Mohr-Coulomb (MC)
The model requires inputting the following parameters: modulus of elasticity E, the Poisson's ratio, angle of internal friction and cohesion. The latter two parameters serve to define the yield condition. The formulation of constitutive equations assumes effective parameters of angle of internal friction φeff and cohesion ceff. The angle of dilation must also be specified.
The Mohr-Coulomb yield surface can be defined in terms of three limit functions that plot as a non-uniform hexagonal cone in the principal stress space. Projections of this yield surface into deviatioric and meridian planes appear in the Figure. As evident from this Figure (part a) the MC yield function has corners, which may cause certain complications in the implementation of this model into the finite element method. The advantage on the other hand is the fact that the traditional soil mechanics and partially also the rock mechanics are based on this model.
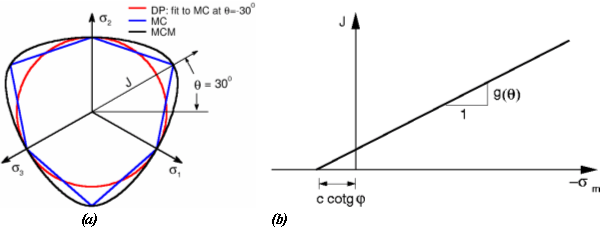 Projection of yield surfaces into: (a) deviatoric, (b) meridian plane
Projection of yield surfaces into: (a) deviatoric, (b) meridian plane